In this section, we look in more detail at some aspects of the kinetic-molecular model and how it relates to our empirical knowledge of gases. For most students, this will be the first application of algebra to the development of a chemical model; this should be educational in itself, and may help bring that subject back to life for you! As before, your emphasis should on understanding these models and the ideas behind them, there is no need to memorize any of the formulas.
Image: Wikimedia Commons
At temperatures above absolute zero, all molecules are in motion. In the case of a gas, this motion consists of straight-line jumps whose lengths are quite great compared to the dimensions of the molecule. Although we can never predict the velocity of a particular individual molecule, the fact that we are usually dealing with a huge number of them allows us to know what fraction of the molecules have kinetic energies (and hence velocities) that lie within any given range.
Many molecules, many velocities
The trajectory of an individual gas molecule consists of a series of straight-line paths interrupted by collisions. What happens when two molecules collide depends on their relative kinetic energies; in general, a faster or heavier molecule will impart some of its kinetic energy to a slower or lighter one. Two molecules having identical masses and moving in opposite directions at the same speed will momentarily remain motionless after their collision.
If we could measure the instantaneous velocities of all the molecules in a sample of a gas at some fixed temperature, we would obtain a wide range of values. A few would be zero, and a few would be very high velocities, but the majority would fall into a more or less well defined range.
We might be tempted to define an "average velocity" for a collection of molecules, but here we would need to be careful: molecules moving in opposite directions have velocities of opposite signs. Because the molecules are in a gas are in random thermal motion, there will be just about as many molecules moving in one direction as in the opposite direction, so the velocity vectors of opposite signs would all cancel and the average velocity would come out to zero. Since this answer is not very useful, we need to do our averaging in a slightly different way.
A single molecule in a gas, moving at a velocity v along an arbitrary axis z, can be moving in the direction +z or –z with equal probability.
... so the conventional "average" velocity
along this axis will be
(+v + –v)/2 = 0
(not very useful!)
We can avoid this dead-end by averaging the square of the velocity: 
This works because (–v)2 = v2 .
Finally, taking the square root of yields the root mean square average 
which we abbreviate as vrms.
We can similarly define the rms average velocity of all the molecules in a gas by the expression
in which the Σ v2 term ("sigma v-squared") denotes the sum of the v2 values for each molecule.
Farther on in this lesson, we will relate vrms to the kinetic energy of gas.
Calculating the RMS average velocity
It is frequently useful to know vrms for a particular gas. Before we do this,
The formula relating the RMS velocity to the temperature and molar mass is surprisingly simple, considering the great complexity of the events it represents:
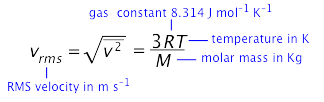
in which m is the molar mass in kg mol–1, and k = R÷6.02E23, the “gas constant per molecule", is known as the Boltzmann constant.
A simpler formula for estimating average molecular velocities is
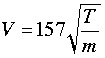
in which V is in units of meters/sec, T is the absolute temperature and m the molar mass in grams.
The Boltzmann distribution
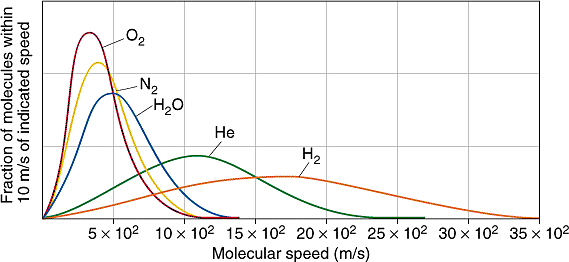
If we were to plot the number of molecules whose velocities fall within a series of narrow ranges, we would obtain a slightly asymmetric curve known as a velocity distribution. The peak of this curve would correspond to the most probable velocity. This velocity distribution curve is known as the Maxwell-Boltzmann distribution, but is frequently referred to only by Boltzmann's name.
The Maxwell-Boltzmann distribution law was first worked out around 1850 by the great Scottish physicist, James Clerk Maxwell (left, 1831-1879), who is better known for disovering the laws of electromagnetic radiation. Later, the Austrian physicist Ludwig Boltzmann (1844-1906)
put the relation on a sounder theoretical basis and simplified the mathematics somewhat. Boltzmann pioneered the application of statistics to the physics and thermodynamics of matter, and was an ardent supporter of the atomic theory of matter at a time when it was still not accepted by many of his contemporaries.
The derivation of the Boltzmann curve is a bit too complicated to go into here, but its physical basis is easy to understand. Consider a large population of molecules having some fixed amount of kinetic energy. As long as the temperature remains constant, this total energy will remain unchanged, but it can be distributed among the molecules in many different ways, and this distribution will change continually as the molecules collide with each other and with the walls of the container.
.
Although the number of possible higher-energy states is greater, the lower-energy states are more likely to be occupied 
. This is because only so much kinetic energy available to the gas as a whole; every molecule that acquires kinetic energy in a collision leaves behind another molecule having less. This tends to even out the kinetic energies in a collection of molecules, and ensures that there are always some molecules whose instantaneous velocity is near zero. The net effect of these two opposing tendencies, one favoring high kinetic energies and the other favoring low ones, is the peaked curve 
seen above. Notice that because of the assymetry of this curve, the mean (rms average) velocity is not the same as the most probable velocity, which is defined by the peak of the curve.
At higher temperatures (or with lighter molecules) the latter constraint becomes less important, and the mean velocity increases. But with a wider velocity distribution, the number of molecules having any one velocity diminishes, so the curve tends to flatten out.
Velocity distributions depend on temperature and mass
Higher temperature broadens the velocity distribution
Higher temperatures allow a larger fraction of molecules to acquire greater amounts of kinetic energy, causing the Boltzmann plots to spread out.
Notice how the left ends of the plots are anchored at zero velocity (there will always be a few molecules that happen to be at rest.) As a consequence, the curves flatten out as the higher temperatures make additional higher-velocity states of motion more accessible. The area under each plot is the same for a constant number of molecules.
Larger molecular weights narrow the velocity distribution
All molecules have the same kinetic energy (mv2/2) at the same temperature; this means that for lighter molecules (smaller m), there must be a larger fraction possessing higher velocities if mv2 is to remain constant. This has the efffect of broadening the distribution of velocities, and at the same time reducing the fraction that have the maximum velocities. Comparison of the distribution plots for O2 and H2 are quite striking in this respect; the fastest molecules of dihydrogen in the earth's atmosphere are able to escape the earth's gravitational field and fly off into space, as is explained farther on
The time-of-flight spectrometer directs a beam of molecules at two rotating sector disks.
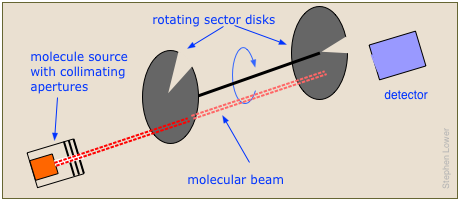
By adjusting the rotational speed and the angular separation between the sectors, only those molecules within a selected range of velocities will reach the detector. The whole experiment must be carried out in a near-vacuum to keep the beam from being dispersed by air molecules.
Boltzmann distribution and planetary atmospheres
The ability of a planet to retain an atmospheric gas depends on the average velocity (and thus on the temperature and mass) of the gas molecules and on the planet's mass, which determines its gravity and thus the escape velocity. In order to retain a gas for the age of the solar system, the average velocity of the gas molecules should not exceed about one-sixth of the escape velocity. The escape velocity from the Earth is 11.2 km/s, and 1/6 of this is about 2 km/s. Examination of the plot of molecular velocities reveals that hydrogen molecules can easily achieve this velocity, and this is the reason that hydrogen, the most abundant element in the universe, is present in only trace amounts in Earth's atmosphere.
Although hydrogen is not a significant atmospheric component, water vapor is. A very small amount of this diffuses to the upper part of the atmosphere, where intense solar radiation breaks down the H2O into H2. Escape of this hydrogen from the upper atmosphere amounts to about 2.5 × 1010 g/year.
As we all know, the fundamental "properties" of a gas are its pressure, volume, and temperature. But if you think about it, only the latter, which determines the average velocity of the particles that constitute the gas, relates directly to the conditions experienced by these particles. The volume of a gas is really a property of the enclosure that confines the gas, while the pressure is a force experienced by the surfaces of that container.
The beauty of the kinetic-molecular model lies in its ability to predict the properties of a perfect gas from nothing more than simple mechanics.
How the K-M theory predicts the pressure of a gas
1 - the force produced when a gas particle collides with a container wall
We begin by recalling that the pressure of a gas arises from the force exerted when molecules collide with the walls of the container. This force can be found from Newton's law
(2-1)
in which v is the velocity component of the molecule in the direction perpendicular to the wall and m is its mass.
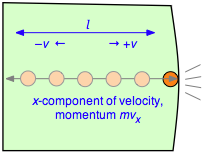
2 - the frequency of collisions
After the collision the molecule must travel a distance l to the opposite wall, and then back across this same distance before colliding again with the wall in question. This determines the time between successive collisions with a given wall; the number of collisions per second will be v÷2l. The force exerted on the wall is the rate of change of the momentum, given by the product of the momentum change per collision and the collision frequency:
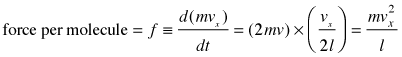(2-2)
Pressure is force per unit area, so the pressure exerted by the molecule on a surface of cross-section l2 becomes
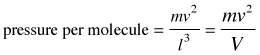(2-3)
in which V is the volume of the box.
3 - the pressure produced by N molecules
We have calculated the pressure due to a single molecule moving at a constant velocity in a direction perpendicular to a wall. If we now introduce more molecules, we must interpret v 2 the root mean square average value which we will denote by, so v 2 becomes .
(2-4)
The temperature of a gas is a measure of the average translational kinetic energy of its molecules, so we begin by calculating the latter.
The translational kinetic energy
Recalling that ½mis the average translational kinetic energy ε, we can rewrite the above expression as
(2-5)
How translational kinetic energy relates to the temperature of a gas
The 2/3 factor in the proportionality reflects the fact that velocity components in each of the three directions contributes ½ kT to the kinetic energy of the particle.
The average translational kinetic energy is directly proportional to temperature:
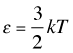(2-6)
in which the proportionality constant k is known as the Boltzmann constant.
Substituting this into (2-5) yields
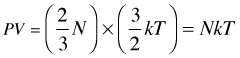(2-7)
K-M theory predicts the ideal gas equation of state
Do you notice that Eq (2-7) looks very much like the ideal gas equation
PV = nRT ? It's not quite the same, however; we have been using capital N to denote the number of molecules, whereas n stands for the number of moles. And of course, the proportionality factor is not the gas constant R, but rather the Boltzmann constant, 1.381 × 10–23 J K–1.
Ah, but it so happens that if we multiply k by Avogadro's number, we get
(1.381 × 10–23 J K–1) (6.022 × 1023) = 8.314 J K–1. In other words, the Boltzmann constant k is just the gas constant per molecule. So for n moles of particles, the Eq. 2-7 turns into our old friend
P V = n R T (2-8)
The ideal gas equation of state came about by combining the empirically determined laws of Boyle, Charles, and Avogadro, but one of the triumphs of the kinetic molecular theory was the derivation of this equation from simple mechanics in the late nineteenth century. This is a beautiful example of how the principles of elementary mechanics can be applied to a simple model to develop a useful description of the behavior of macroscopic matter, and it will be worth your effort to follow and understand the individual steps of the derivation. (But don't bother to memorize it!)
RT has the dimensions of energy
Since the product PV has the dimensions of energy, so does RT, and this quantity in fact represents the average translational kinetic energy per mole of molecular particles. The relationship between these two energy units can be obtained by recalling that 1 atm is 1.013E5 N m–2, so that
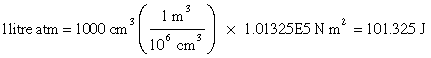
The gas constant R is one of the most important fundamental constants relating to the macroscopic behavior of matter. It is commonly expressed in both pressure-volume and in energy units:
R = 0.082057 L atm mol–1 K–1 = 8.314 J mol–1 K–1
That is, R expresses the amount of energy per Kelvin degree.
As noted above, the Boltzmann constant k, which appears in many expressions relating to the statistical treatment of molecules, is just
R ÷ 6.02E23 = 1.3807 × 10–23 J K–1,
the "gas constant per molecule "
Molecular velocities tend to be very high by our everyday standards (typically around 500 metres per sec), but even in gases, they bump into each other so frequently that their paths are continually being deflected in a random manner, so that the net movement (diffusion) of a molecule from one location to another occurs rather slowly.
The average distance a molecule moves between such collisions is called the mean free path. This distance, denoted by λ (lambda), depends on the number of molecules per unit volume and on their size. To avoid collision, a molecule of diameter σ must trace out a path corresponding to the axis of an imaginary cylinder whose cross-section is πσ2. Eventually it will encounter another molecule (extreme right in the diagram below) that has intruded into this cylinder and defines the terminus of its free motion.
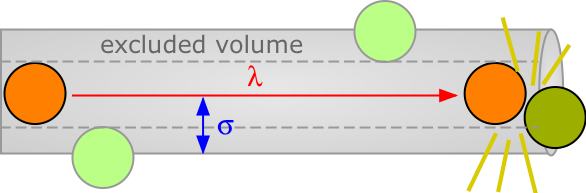
The volume of the cylinder is πσ2/λ. At each collision the molecule is diverted to a new path and traces out a new exclusion cylinder. After colliding with all n molecules in one cubic centimetre of the gas it will have traced out a total exclusion volume of πσ2λ. Solving for λ and applying a correction factor √2 to take into account exchange of momentum between the colliding molecules (the detailed argument for this is too complicated to go into here), we obtain
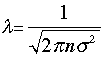(3-1)
Small molecules such as He, H2 and CH4 typically have diameters of around 30-50 pm. At STP the value of n, the number of molecules per cubic metre, is
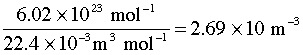
Substitution into (3-1) yields a value of around 10–7 m (100 nm) for the mean free path of most molecules under these conditions. Although this may seem like a very small distance, it typically amounts to 100 molecular diameters, and more importantly, about 30 times the average distance between molecules. This explains why so many gases conform very closely to the ideal gas law at ordinary temperatures and pressures.
On the other hand, at each collision the molecule can be expected to change direction. Because these changes are random, the net change in location a molecule experiences during a period of one second is typically rather small. Thus in spite of the high molecular velocities, the speed of molecular diffusion in a gas is usually quite small.
