Moles, mixtures and densities of gases
Dalton's law of partial pressures and the E.V.E.N. principle
Although all gases closely follow the ideal gas law PV = nRT under appropriate conditions, each gas is also a unique chemical substance consisting of molecular units that have definite masses. In this lesson we will see how these molecular masses affect the properties of gases that conform to the ideal gas law.
Following this, we will look at gases that contain more than one kind of molecule— in other words, mixtures of gases.
We begin with a review of molar volume and the E.V.E.N. principle, which is central to our understanding of gas mixtures.
You will recall that the molar mass of a pure substance is the mass of 6.02 x 1023 (Avogadro's number) of particles or molecular units of that substance. Molar masses are commonly expressed in units of grams per mole (g mol–1) and are often referred to as molecular weights.
As was explained in the preceding lesson, equal volumes of gases, measured at the same temperature and pressure, contain equal numbers of molecules (this is the "EVEN" principle, more formally known as Avogadro's law.)
Standard temperature and pressure: 273K, 1 atm
This means that one mole of any gas will occupy the same volume as one mole of any other gas at a given temperature and pressure. The magnitude of this volume will of course depend on the temperature and pressure, so as a means of convenient comparison it is customary to define a set of conditions T = 273K and P = 1 atm as standard temperature and pressure, usually denoted as STP. Substituting these values into the ideal gas equation of state and solving for V yields a volume of 22.414 litres for 1 mole.
The standard molar volume 22.4 L mol –1 is a value worth memorizing, but remember that it is valid only at STP. The molar volume at other temperatures and pressures can easily be found by simple proportion.
The molar volume of a substance can tell us something about how much space each molecule occupies, as the following example shows.
Pure gases or mixtures: it makes no difference
Under conditions at which the ideal gas model is applicable (that is, almost always unless you are a chemical engineer dealing with high pressures), "a molecule is a molecule", so the volume of Avogadro's number of molecules will be independent of the composition of the gas. The reason, of course, is that the volume of the gas is mostly empty space; the volumes of the molecules themselves are negligible.
The molecular weight (molar mass) of any gas is the mass, expressed in grams, of Avogadro's number of its molecules. This is true regardless of whether the gas is composed of one molecular species or is a mixture.
For a mixture of gases, the molar mass will depend on the molar masses of its components, and on the fractional abundance of each kind of molecule in the mixture. The term "average molecular weight" is often used to describe the molar mass of a gas mixture.
Average molecular weight of a gas
The average molar mass![]() of a mixture of gases is just the sum of the mole fractions xi of each gas, multiplied by the molar mass mi of that substance:
of a mixture of gases is just the sum of the mole fractions xi of each gas, multiplied by the molar mass mi of that substance:
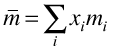
Density of a pure gas
The molar volumes of all gases are the same when measured at the same temperature and pressure. But the molar masses of different gases will vary. This means that different gases will have different densities (different masses per unit volume). If we know the molecular weight of a gas, we can calculate its density.
More importantly, if we can measure the density of an unknown gas, we have a convenient means of estimating its molecular weight. This is one of many important examples of how a macroscopic measurement (one made on bulk matter) can yield microscopic information (that is, about molecular-scale objects.)
Determination of the molecular weight of a gas from its density is known as the Dumas method, after the French chemist Jean Dumas (1800-1840) who developed it. One simply measures the weight of a known volume of gas and converts this volume to its STP equivalent, using Boyle's and Charles' laws. The weight of the gas divided by its STP volume yields the density of the gas, and the density multiplied by 22.4 mol–1 gives the molecular weight. Pay careful attention to the examples of gas density calculations shown here and in your textbook. You will be expected to carry out calculations of this kind, converting between molecular weight and gas density.
Because most of the volume occupied by a gas consists of empty space, there is nothing to prevent two or more kinds of gases from occupying the same volume. Homogeneous mixtures of this kind are generally known as solutions, but it is customary to refer to them simply as gaseous mixtures.
We can specify the composition of gaseous mixtures in many different ways, but the most common ones are by volumes and by mole fractions.
Volume fractions in gas mixtures
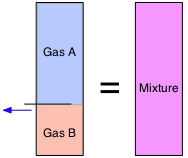
We can specify the composition of this mixture by saying that the volume fraction of A is 2/3 and that of B is 1/3.
Volume fractions are often called partial volumes:
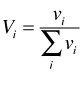
Don't let this type of notation put you off! The summation sign Σ (Greek Sigma) simply means to add up the v's (volumes) of every gas. Thus if Gas A is the "i-th" substance as in the expression immediately above, the summation runs from i=1 through i=2.
Note that we can employ partial volumes to specify the composition of a mixture even if it had never actually been made by combining the pure gases.
When we say that air, for example, is 21 percent oxygen and 78 percent nitrogen by volume, this is the same as saying that these same percentages of the molecules in air consist of O2 and N2. Similarly, in 1.0 mole of air, there is 0.21 mol of O2 and 0.78 mol of N2 (the other 0.1 mole consists of various trace gases, but is mostly neon.)
Note that you could never assume a similar equivalence with mixtures of liquids or solids, to which the E.V.E.N. principle does not apply.
Mole fractions in gas mixtures
These last two numbers (.21 and .78) also express the mole fractions of oxygen and nitrogen in air. Mole fraction means exactly what it says: the fraction of the molecules that consist of a specific substance. This is expressed algebraically by
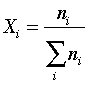
so in the case of oxygen in the air, its mole fraction is

Problem Example 7
A mixture of O2 and nitrous oxide, N2O, is sometimes used as a mild anesthetic in dental surgery. A certain mixture of these gases has a density of 1.482 g L–1 at 25 and 0.980 atm. What was the mole-percent of N2O in this mixture?
Solution: First, find the density the gas would have at STP:
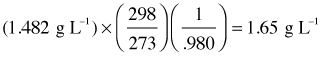
The molar mass of the mixture is (1.65 g L–1)(22.4 L mol–1) = 37.0 g mol–1. The molecular weights of O2 and N2 are 32 and 44, respectively. 37.0 is 5/12 of the difference between the molar masses of the two pure gases. Since the density of a gas mixture is directly proportional to its average molar mass, the mole fraction of the heavier gas in the mixture is also 5/12:
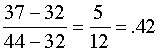
Problem Example 8
What is the mole fraction of carbon dioxide in a mixture consisting of equal masses of CO2 (MW=44) and neon (MW=20.2)?
Solution: Assume any arbitrary mass, such as 100 g, find the equivalent numbers of moles of each gas, and then substitute into the definition of mole fraction:
nCO2 = (100 g) ÷ (44 g mol–1) = 2.3 mol
nNe= (100 g) ÷ (20.2 g mol–1) = 4.9 mol
XNe = (2.3 mol) ÷ (2.3 mol + 4.9 mol) = 0.32
 The ideal gas equation of state applies to mixtures just as to pure gases. It was in fact with a gas mixture, ordinary air, that Boyle, Gay-Lussac and Charles did their early experiments. The only new concept we need in order to deal with gas mixtures is the partial pressure, a concept invented by the famous English chemist John Dalton (1766-1844). Dalton reasoned that the low density and high compressibility of gases indicates that they consist mostly of empty space; from this it follows that when two or more different gases occupy the same volume, they behave entirely independently.
The ideal gas equation of state applies to mixtures just as to pure gases. It was in fact with a gas mixture, ordinary air, that Boyle, Gay-Lussac and Charles did their early experiments. The only new concept we need in order to deal with gas mixtures is the partial pressure, a concept invented by the famous English chemist John Dalton (1766-1844). Dalton reasoned that the low density and high compressibility of gases indicates that they consist mostly of empty space; from this it follows that when two or more different gases occupy the same volume, they behave entirely independently.
The contribution that each component of a gaseous mixture makes to the total pressure of the gas is known as the partial pressure of that gas. Dalton himself stated this law in the simple and vivid way shown at the left.
The usual way of stating Dalton's Law of Partial Pressures is
The total pressure of a gas is the sum
of the partial pressures of its components
We can express this algebraically as
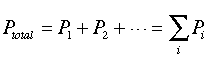
or, equivalently
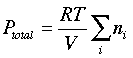
(If you feel a need to memorize these formulas, you probably don't really understand Dalton's Law!)
There is also a similar relationship based on volume fractions, known as Amagat's law of partial volumes. It is exactly analogous to Dalton's law, in that it states that the total volume of a mixture is just the sum of the partial volumes of its components. But there are two important differences: Amagat's law holds only for ideal gases which must all be at the same temperature and pressure. Dalton's law has neither of these restrictions.
Although Amagat's law seems intuitively obvious, it sometimes proves useful in chemical engineering applications. We will make no use of it in this course.
Problem Example 9
Calculate the mass of each component present in a mixture of fluorine (MW and xenon (MW 131.3) contained in a 2.0-L flask. The partial pressure of Xe is 350 torr and the total pressure is 724 torr at 25°C.
Solution: From Dalton's law, the partial pressure of F2 is (724 – 350) = 374 torr:
The mole fractions are XXe = 350/724 = .48 and XF2 = 374/724 = .52 . The total number of moles of gas is
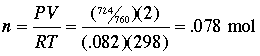
The mass of Xe is (131.3 g mol–1) × (.48 × .078 mol) = 4.9 g
Problem Example 10
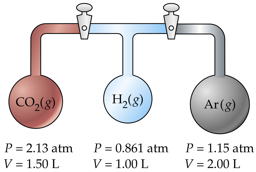
Three flasks having different volumes and containing different gases at various pressures are connected by stopcocks as shown. When the stopcocks are opened,
a) What will be the pressure in the system?
b) Which gas will be most abundant in the mixture?
Assume that the temperature is uniform and that the volume of the connecting tubes is negligible.
Solution: The trick here is to note that the total number of moles nT and the temperature remain unchanged, so we can make use of Boyle's law PV = constant. We will work out the details for CO2 only, denoted by subscripts a.
For CO2, PaVa = (2.13 atm)(1.50 L) = 3.19 L-atm.
Adding the PV products for each separate container, we obtain
ΣPiVi = 6.36 L-atm = nT RT. We will call this sum P1V1.
After the stopcocks have been opened and the gases mix, the new conditions are denoted by P2V2.
From Boyle's law, P1V1 = P2V2 = 6.36 L-atm. V2 = ΣVi = 4.50 L.
Solving for the final pressure P2 we obtain (6.36 L-atm)/(4.50 L) = 1.41 atm.
For part (b), note that the number of moles of each gas is n = PV/RT. The mole fraction if any one gas is Xi = ni /nT . For CO2, this works out to
(3.19/RT) / (6.36/RT) = 0.501. Because this exceeds 0.5, we know that this is the most abundant gas in the final mixture.
Collecting gases over water
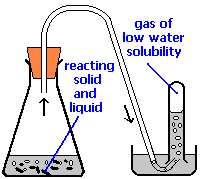 A common laboratory method of collecting the gaseous product of a chemical reaction is to conduct it into an inverted tube or bottle filled with water, the opening of which is immersed in a larger container of water. This arrangement is called a pneumatic trough, and was widely used in the early days of chemistry. As the gas enters the bottle it displaces the water and becomes trapped in the upper part.
A common laboratory method of collecting the gaseous product of a chemical reaction is to conduct it into an inverted tube or bottle filled with water, the opening of which is immersed in a larger container of water. This arrangement is called a pneumatic trough, and was widely used in the early days of chemistry. As the gas enters the bottle it displaces the water and becomes trapped in the upper part.
The volume of the gas can be observed by means of a calibrated scale on the bottle, but what about its pressure? The total pressure confining the gas is just that of the atmosphere transmitting its force through the water. (An exact calculation would also have to take into account the height of the water column in the inverted tube.) But liquid water itself is always in equilibrium with its vapor, so the space in the top of the tube is a mixture of two gases: the gas being collected, and gaseous H2O. The partial pressure of H2O is known as the vapor pressure of water and it depends on the temperature. In order to determine the quantity of gas we have collected, we must use Dalton's Law to find the partial pressure of that gas.
Problem Example 11
Oxygen gas was collected over water as shown above. The atmospheric pressure was 754 torr, the temperature was 22°C, and the volume of the gas was 155 mL. The vapor pressure of water at 22°C is 19.8 torr. Use this information to estimate the number of moles of O2 produced.
Solution:
From Dalton's law, PO2= Ptotal – PH2O = 754 – 19.8 = 734 torr = .966 atm.
Scuba diving and Dalton's law
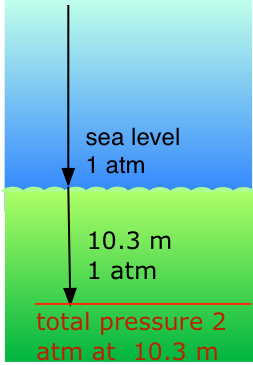
 Our respiratory systems are designed to maintain the proper oxygen concentration in the blood when the partial pressure of O2 is 0.21 atm, its normal sea-level value. Below the water surface, the pressure increases by 1 atm for each 10.3 m increase in depth; thus a scuba diver at 10.3 m experiences a total of 2 atm pressure pressing on the body. In order to prevent the lungs from collapsing, the air the diver breathes should also be at about the same pressure.
Our respiratory systems are designed to maintain the proper oxygen concentration in the blood when the partial pressure of O2 is 0.21 atm, its normal sea-level value. Below the water surface, the pressure increases by 1 atm for each 10.3 m increase in depth; thus a scuba diver at 10.3 m experiences a total of 2 atm pressure pressing on the body. In order to prevent the lungs from collapsing, the air the diver breathes should also be at about the same pressure.
But at a total pressure of 2 atm, the partial pressure of O2 in ordinary air would be 0.42 atm; at a depth of 100 ft (about 30 m), the O2 pressure of .8 atm would be sufficiently high to induce oxygen toxicity. For this reason, the air mixture in the pressurized tanks that scuba divers wear must contain a smaller fraction of O2. This can be achieved most simply by raising the nitrogen content, but high partial pressures of N2 can also be dangerous, resulting in a condition known as nitrogen narcosis. The preferred diluting agent for sustained deep diving is helium, which has very little tendency to dissolve in the blood even at high pressures.
Make sure you thoroughly understand the following essential ideas which have been presented above. It is especially important that you know the precise meanings of all the highlighted terms in the context of this topic.
- One mole of a gas occupies a volume of 22.4 L at STP (standard temperature and pressure, 273K, 1 atm = 103 kPa.)
- The above fact allows us to relate the measurable property of the density of a gas to its molar mass.
- The composition of a mixture of gases is commonly expressed in terms mole fractions; be sure you know how to calculate them.
- Dalton's Law of partial pressures says that every gas in a mixture acts independently, so the total pressure a gas exerts against the walls of a container is just the sum of the partial pressures of the individual components.