Thermochemistry and calorimetry

In order to define the thermochemical properties of a process, it is first necessary to write a thermochemical equation that defines the actual change taking place, both in terms of the formulas of the substances involved and their physical states (temperature, pressure, and whether solid, liquid, or gaseous.

To take a very simple example, here is the complete thermochemical equation for the vaporization of water at its normal boiling point:

H₂O(l, 373 K, 1 atm) → H₂O(g, 373 K, 1 atm)    ΔH = 40.7 kJ mol^–1

The quantity 40.7 is known as the enthalpy of vaporization (often referred to as “heat of vaporization”) of liquid water.

It is essential that the following points be kept in mind when writing thermochemical equations:

• Any thermodynamic quantity such as ΔH that is associated with a thermochemical equation always refers to the number of moles of substances explicitly shown in the equation.

Thus for the synthesis of water we can write

2 H₂(g) + O₂(g)→ 2 H₂O(l)    ΔH = –572 kJ

or

H₂(g) + ½ O₂(g)→ H₂O(l)    ΔH = –286 kJ

• Thermochemical equations for reactions taking place in solution must also specify the concentrations of the dissolved species.

For example, the enthalpy of neutralization of a strong acid by a strong base is given by

in which the abbreviation aq refers to the hydrated ions as they exist in aqueous solution. Since most thermochemical equations are written for the standard conditions of 298 K and 1 atm pressure, we can leave these quantities out if these conditions apply both before and after the reaction. If, under these same conditions, the substance is in its preferred (most stable) physical state, then the substance is said to be in its standard state.

Thus the standard state of water at 1 atm is the solid below 0°C, and the gas above 100°C. A thermochemical quantity such as ΔH that refers to reactants and products in their standard states is denoted by ΔH°.

• In the case of dissolved substances, the standard state of a solute is that in which the “effective concentration”, known as the activity, is unity.

For non-ionic solutes the activity and molarity are usually about the same for concentrations up to about 1M, but for an ionic solute this approximation is generally valid only for solutions more dilute than 0.001-0.01M, depending on electric charge and size of the particular ion.

The enthalpy change for a chemical reaction is the difference

ΔH = H_products – H_reactants

If the reaction in question represents the formation of one mole of the compound from its elements in their standard states.

H₂(g) + ½ O₂(g)→ H₂O(l)    ΔH = –286 kJ

then we can arbitrarily set the enthalpy of the elements to zero and write

H °_reaction = Σ ΔH_f °_products – Σ ΔH_f °_reactants= –286 kJ – 0 = –268 kJ mol^–1

which defines the standard enthalpy of formation of water at 298K.

The value H_f ° = –268 kJ tells us that when hydrogen and oxygen, each at a pressure of 1 atm and at 298 K (25° C) react to form 1 mole of liquid water also at 25°C and 1 atm pressure, 268 kJ will have passed from the sytstem (the reaction mixture) into the surroundings. The negative sign indicates that the reaction is exothermic: the enthalpy of the product is smaller than that of the reactants.

The standard enthalpy of formation is a fundamental property of any compound. Most chemistry textbooks contain tables of H_f ° values (usually alongside values of other thermodynamic properties) in their appendices. There are also many Web-based sources, such as the one at chemistry.about.com.

In general, the standard enthalpy change for a reaction is given by the expression

important ⇒ΔH°_reaction = Σ ΔH_f °_products Σ ΔH_f °_reactants (2-1)

in which the ΣH_f ° terms indicate the sums of the standard enthalpies of formations of all products and reactants. The above definition is one of the most important in chemistry because it allows us to predict the enthalpy change of any reaction without knowing any more than the standard enthalpies of formation of the products and reactants, which are widely available in tables.

The following examples illustrate some other important aspects of the standard enthalpy of formation of substances.

• The thermochemical equation defining H_f ° is always written in terms of one mole of the substance in question:

½ N₂(g) + 3/2 H₂(g)→ NH₃(g)    ΔH° = –46.1 kJ (per mole of NH₃)

•The standard heat of formation of a compound is always taken in reference to the forms of the elements that are most stable at 25°C and 1 atm pressure.

A number of elements, of which sulfur and carbon are common examples, can exist in more then one solid crystalline form.

In the case of carbon, the graphite modification is the more stable form.

C(graphite) + O₂(g) → CO₂(g)    ΔH° ≡ H_f ° = –393.5 kJ mol^–1

C(diamond) + O₂(g) → CO₂(g)    ΔH° = –395.8 kJ mol^–1

• The physical state of the product of the formation reaction must be indicated explicitly if it is not the most stable one at 25°C and 1 atm pressure:

Subtraction of the second reaction from the first (i.e., writing the second equation in reverse and adding it to the first one) yields

C(graphite) → C(diamond)     ΔH° = 1.89 kJ mol^–1

This principle, known as Hess’ law of independent heat summation is a direct consequence of the enthalpy being a state function. Hess’ law is one of the most powerful tools of chemistry, for it allows the change in the enthalpy (and in other thermodynamic functions) of huge numbers of chemical reactions to be predicted from a relatively small base of experimental data.

Because most substances cannot be prepared directly from their elements, heats of formation of compounds are seldom determined by direct measurement. Instead, Hess’ law is employed to calculate enthalpies of formation from more accessible data. The most important of these are the standard enthalpies of combustion. Most elements and compounds combine with oxygen, and many of these oxidations are highly exothermic, making the measurement of their heats relatively easy.

For example, by combining the heats of combustion of carbon, hydrogen, and methane, we can obtain the standard enthalpy of formation of methane, which as we noted above, cannot be determined directly.

More on calorimetry: ChemEd digital library

How are enthalpy changes determined experimentally? First, you must understand that the only thermal quantity that can be observed directly is the heat q that flows into or out of a reaction vessel, and that q is numerically equal to ΔH° only under the special condition of constant pressure. Moreover, q is equal to the standard enthalpy change only when the reactants and products are both at the same temperature, normally 25°C.

The measurement of q is generally known as calorimetry.

The most common types of calorimeters contain a known quantity of water which absorbs the heat released by the reaction. Because the specific heat capacity of water (4.184 J g^–1 K^–1) is known to high precision, a measurement of its temperature rise due to the reaction enables one to calculate the quantity of heat released.

In all but the very simplest calorimeters, some of the heat released by the reaction is absorbed by the components of the calorimeter itself. It is therefore necessary to "calibrate" the calorimeter by measuring the temperature change that results from the introduction of a known quantity of heat. The resulting calorimeter constant, expressed in J K^–1, can be regarded as the “heat capacity of the calorimeter”. The known source of heat is usually produced by passing a known quantity of electric current through a resistor within the calorimeter, but it can be measured by other means as described in the following problem example.

For reactions that can be initiated by combining two solutions, the temperature rise of the solution itself can provide an approximate value of the reaction enthalpy if we assume that the heat capacity of the solution is close to that of the pure water — which will be nearly true if the solutions are dilute. 

videos on Calorimetry

Basics UTexasAustin (5 min);

Bergmann/Sams demos:
  Demo 1/2 (7 min);
  Demo 2/2 (9 min);
  Calorimetry of a Cheeto (9 min);

Heat of solution (rbOttawa,4 m);
Bomb calorimeter (FunOfCombustion, 9 m);
Bomb calculations
(UTexasAustin, 9 min)

For reactions that cannot be carried out in dilute aqueous solution, the reaction vessel is commonly placed within a larger insulated container of water. During the reaction, heat passes between the inner and outer containers until their temperatures become identical. Again, the temperature change of the water is observed, but in this case we need to know the value of the calorimeter constant described above.

The bomb calorimeter

>

Most serious calorimetry carried out in research laboratories involves the determination of heats of combustion, since these are essential to the determination of standard enthalpies of formation of the thousands of new compounds that are prepared and characterized each month.

In order to ensure complete combustion, the experiment is carried out in the presence of oxygen above atmospheric pressure. This requires that the combustion be confined to a fixed volume.

Since the process takes place at constant volume, the reaction vessel must be constructed to withstand the high pressure resulting from the combustion process, which amounts to a confined explosion. The vessel is usually called a “bomb”, and the technique is known as bomb calorimetry. The reaction is initiated by discharging a capacitor through a thin wire which ignites the mixture.

Another consequence of the constant-volume condition is that the heat released corresponds to q_v , and thus to the internal energy change ΔU rather than to ΔH. The enthalpy change is calculated  according to the formjula

ΔH = q_v + Δn_gRT

in which Δn_g  is the change in the number of moles of gases in the reaction.

(See the second lesson in this set for the derivation of this equation).

 

How do calorimeters work?

Although calorimetry is simple in principle, its practice is a highly exacting art, especially when applied to processes that take place slowly or involve very small heat changes, such as the germination of seeds.

How to use a coffee-cup calorimeter (Dartmouth College)

Simple interactive exercise (ChemPages)

Calorimeters can be as simple as a foam plastic coffee cup, which is often used in student laboratories.

 

Research-grade calorimeters, able to detect minute temperature changes, are more likely to occupy table tops, or even entire rooms:

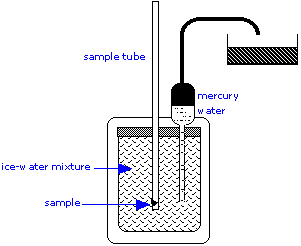The ice calorimeter is an important tool for measuring the heat capacities of liquids and solids, as well as the heats of certain reactions. This simple yet ingenious apparatus is essentially a device for measuring the change in volume due to melting of ice. To measure a heat capacity, a warm sample is placed in the inner compartment, which is surrounded by a mixture of ice and water.

The heat withdrawn from the sample as it cools causes some of the ice to melt. Since ice is less dense than water, the volume of water in the insulated chamber decreases. This causes an equivalent volume of mercury to be sucked into the inner reservoir from the outside container. The loss in weight of this container gives the decrease in volume of the water, and thus the mass of ice melted. This, combined with the heat of fusion of ice, gives the quantity of heat lost by the sample as it cools to 0°C.